Einstein’s Real Equation
Mass, Energy and the Curvature of Space-Time
Sean Carroll
Homewood Professor of Natural Philosophy
Bloomberg Center for Physics and Astronomy
Johns Hopkins University
Sponsored by the IP Law Firm of MWZB, PC
About the Lecture
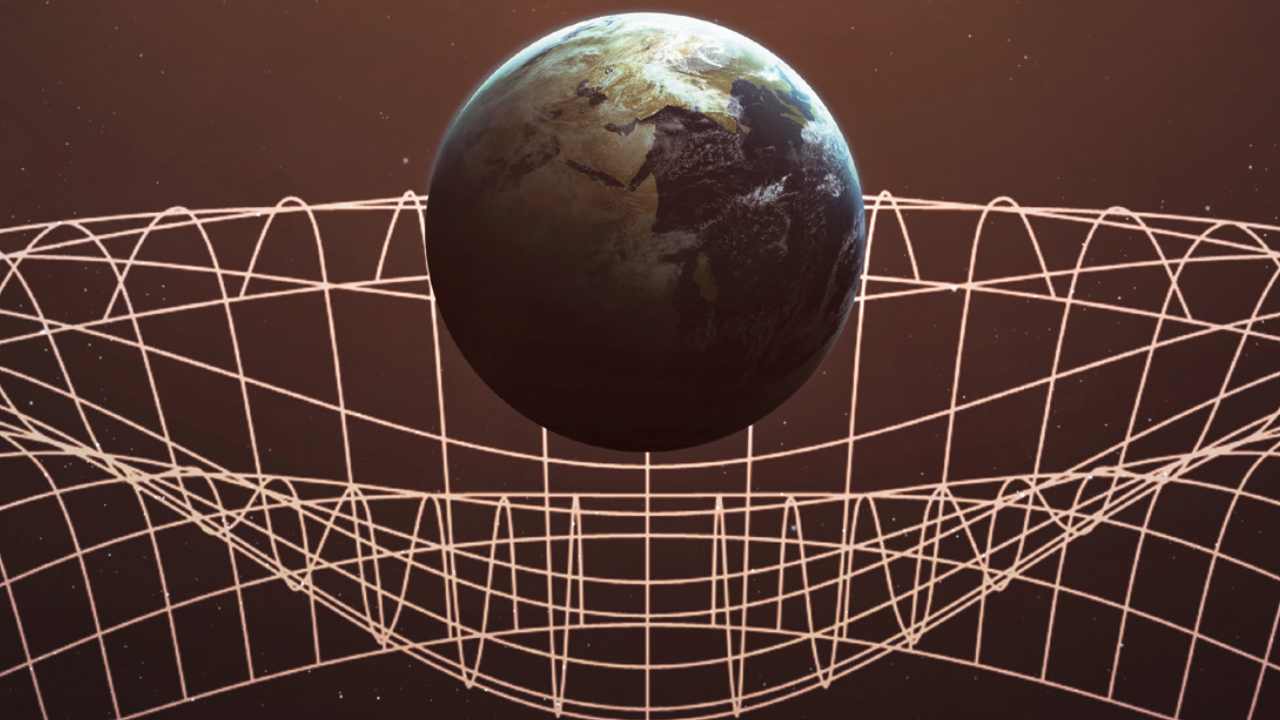
Albert Einstein is indelibly associated with a famous equation: E=mc2, relating the mass of an object to its energy. But that is not what physicists have in mind when they say “Einstein’s Equation.” The “real” Einstein’s Equation – the one physicists have in mind – is part of general relativity, which relates the curvature of spacetime to the mass and energy distributed within it. This lecture will explain why the geometry of spacetime has anything to do with gravity, and how the Einstein Equation expresses how spacetime curves.
Selected Reading & Media References
Sean Carroll, The Biggest Ideas in the Universe: Space, Time, and Motion; Dutton 2022
Sean Carroll, Spacetime and Geometry, An Introduction to General Relativity, Pearson Education – Addison Wesley, San Francisco, 2004
Misner, Throne and Wheeler, Gravitation, Princeton University Press, 2017
About the Speaker

Sean Carroll is Homewood Professor of Natural Philosophy in the Department of Philosophy and Professor in the Department of Physics and Astronomy at Johns Hopkins University, and he is a member of the Fractal Faculty at the Santa Fe Institute. Previously he was Research Professor at Caltech and before that he was on the faculty at the University of Chicago.
Sean is well known for his work in theoretical physics and the philosophy of science. His theoretical work focuses on the foundations of physics, including issues in quantum mechanics and spacetime, and on cosmology. His research publications include work on Lorentz invariance, closed time-like curves in general relativity, topological defects in field theory; extra spacetime dimensions, dark energy and its interactions with ordinary matter and dark matter, and on modifications of general relativity and aspects of quantum mechanics, including the many-worlds interpretation and a derivation of the Born rule for probabilities.
Sean is an author on many technical and general publications and books, including the well-received textbook Spacetime and Geometry, An Introduction to General Relativity and his most recent book is The Biggest Ideas in the Universe, Vol. 1: Space, Time, and Motion. Sean also has made two lecture series on physics on Wondrium/The Great Courses. And he hosts Mindscape, a weekly podcast.
Among many other honors and awards, Sean has been awarded prizes and fellowships by the National Science Foundation, NASA, the Sloan Foundation, the Packard Foundation, the American Physical Society, the American Institute of Physics, the Royal Society of London, the Guggenheim Foundation, and the American Association for the Advancement of Science.
Sean earned a BS in Astronomy and Astrophysics with a minor in Philosophy at Villanova University and a PhD in Astronomy at Harvard University.
Minutes
On June 2, 2023, from the Powell Auditorium of the Cosmos Club in Washington, D.C, and by live broadcast on the PSW Science YouTube channel, President Larry Millstein called the 2,478th meeting of the Society to order at 8:00 p.m. ET. He welcomed new members, and the recording secretary read the minutes of the previous meeting.
President Millstein then introduced the speaker for the evening, Sean Carroll, The Homewood Professor of Natural Philosophy in the Department of Philosophy and Professor in the Department of Physics and Astronomy at Johns Hopkins University. His lecture was titled, “Einstein’s Real Equation: Mass, Energy and the Curvature of Space-Time.”
He began by expressing excitement about being back on the East coast, a place where the idea of an exciting thing to do on a Friday night is to go to a physics lecture. Carroll then explained that the lecture was inspired from his recently published book, though it is not a talk about the book. He then went on to review the famous Einstein’s Equations, E=mc^2, and the real Einstein equation, which he would spend the remainder of the lecture discussing.
The speaker continued in what he referred to as first gear, with some fundamental equations upon which his argument would build. The equations reviewed were that of Newton's second law of motion, and Newton's inverse square law. He emphasized that all objects accelerate the same under the force of gravity.
The speaker then went into second gear by reviewing the history of Einstein’s thought processes and how he could not reconcile electromagnetism and gravity. The speaker introduced a mathematician and one of Einsteins professors, Herman Minkowski, and his desire and accomplishment to unify space and time mathematically. Carroll then went into a lengthy math argument enabling the intuitive understanding of Minkowski’s accomplishment of making Einstein's theory more beautiful.
Carroll then went into third gear by shifting to explain the geometry of curved space time. Carroll reviewed Euclidean geometry, as based on the parallel postulate theorem, and Reimann geometry, developed by the genius mathematician Bernard Reimann.
The fourth gear argument, Carroll described next, was the difference between Euclidean space and Minkowski spacetime. Once again Carroll explained these highly mathematical concepts in an intuitive manner.
He then introduced tensors in a digestible manner and gave an anecdote on the popular film, Intersteller, to keep the audience slightly amused. Carroll explained that Physicist Kip Thorne worked with the film's special effects wizards and wrote a peer-reviewed publication on how to depict a black hole for purposes of showing in the movie.
For the fifth gear discussion, the speaker reviewed that gravity is the curvature of spacetime, and the curvature of spacetime is captured by the Reimann tensor. He went on to explain how gravity could be turned into a statement about the Riemann tensor. The mathematical difficulty, he explained, was in the fundamental difference between the vector types; however, when Einstein resolved this issue, he had come up with a way to set the curvature of spacetime equal to the energy momentum tensor, or Einstein’s equation.
Carroll next displayed a single component of the Reimann tensor as a demonstration of how difficult Einstein’s equation was to solve. Kids these days, he lightheartedly complained, don’t know what it’s like to pull an all nighter to calculate this!
The speaker explained that Einstein had only ever approximately solved his equation and that the first person to solve Einstein’s equation was a German mathematician at the Eastern front during World War One named Karl Schwarzschild. He found a solution with a feature at R = 2GM. This would later be known as the Schwarzschild radius and marks the event horizon.
A question from a member in the audience asked why we do not observe a train wreck of stuff accumulated at the edge of a black hole. The speaker responded with, I lied, to which the audience laughed. Carroll went on to explain that the objects falling into a black hole add to the gravitational field because they have matter and energy, so the event horizon expands to consume the newly acquired objects. So what we actually observe is objects moving infinitely slower and slower then they are in fact swallowed up by the black hole. Another member in the audience asked about the relationship between Einstein’s special relativity and general relativity. The speaker explained that general relativity is not in fact a generalized version of special relativity and that special relativity is a hundred percent compatible with acceleration and accelerated frames of references.
After the question-and-answer period, President Millstein thanked the speaker, made the usual housekeeping announcements, and invited guests to join the Society. President Millstein adjourned the meeting at 9:48 p.m.
Temperature in Washington, D.C.: 20° C
Weather: Partly Cloudy
Attendance in person in the Powell Auditorium: 98
Number of streaming online viewers, 71, for a total in-person attendance of 169.
Views during the 2-week period after the lecture: 40,046
Respectfully submitted,
Cameo Lance, Recording Secretary